Example 1: By Hand

Given $y=3x^2–9x+13$, determine ${\frac{\mathit{dy}}{\mathit{dx}}|}_{x=3}$.

Step 1: Find the Derivative

This derivative requires us to apply the power rule.

$y=3x^2–9x+13$

$\frac{\mathit{dy}}{\mathit{dx}}=6x–9$

Step 2: Plug the given value into the equation.

In this example we are being asked to plug 3 in for x .

${\frac{\mathit{dy}}{\mathit{dx}}|}_{x=3}=6\left(3\right)–9$

${\frac{\mathit{dy}}{\mathit{dx}}|}_{x=3}=\mathit{9}$

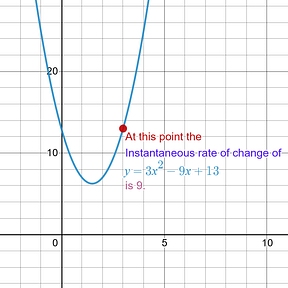
Final Result:

The value of the derivative, $\frac{\mathit{dy}}{\mathit{dx}}$, of the function $y=3x^2–9x+13$ at x = 3 is 9 .

Meaning:

–           The instantaneous rate of change of $y=3x^2–9x+13$ at x = 3 is 9 .

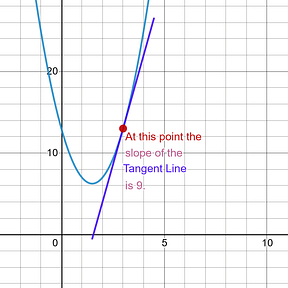
–           The slope of $y=3x^2–9x+13$at x = 3 is 9 .

–           The slope of the tangent line to $y=3x^2–9x+13$ at x = 3 is 9 .